10 Examples of Parabolic Motion

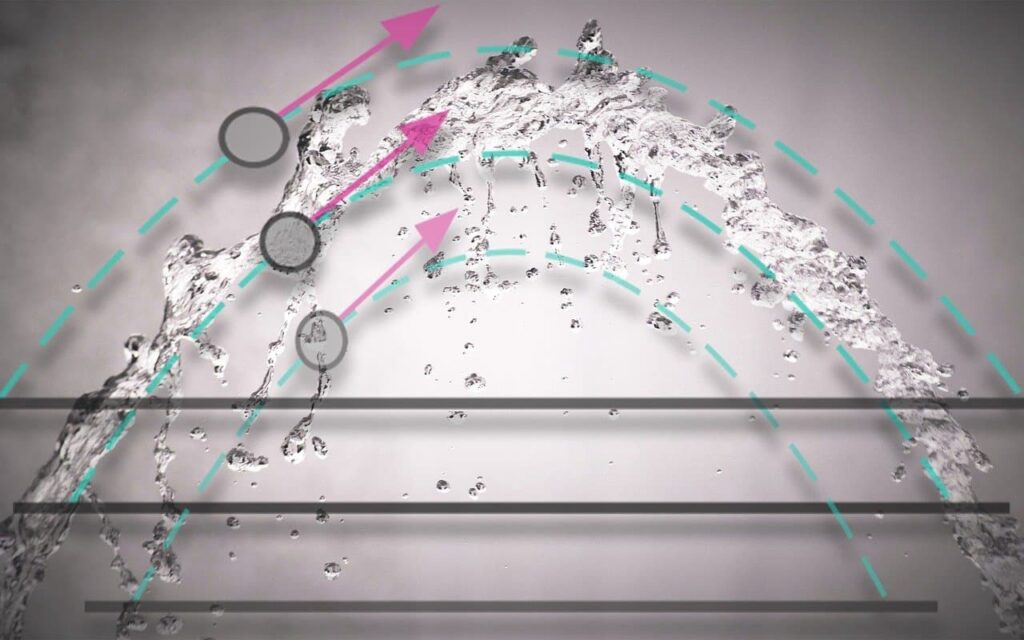
It is called parabolic motion or parabolic shot the displacement of an object whose path traces the shape of a parabola.

The parabolic movement is characteristic of an object or projectile subject to the laws of a uniform gravitational field that crosses a medium of little or no resistance and is considered the conjunction of two different movements simultaneously: a uniform horizontal displacement and other accelerated vertical.

It is the movement of any object that is thrown with a speed that has a component parallel to the earth’s surface and another perpendicular. The thrown objects would trace an ellipse with one of their foci in the gravitational center of our planet if it weren’t for the fact that they find the ground before they can. Thus, its path is finally that of an ellipse segment, coinciding with a parabola.

For this reason, the formulas of the parabola are used to calculate this type of movement.

In addition, the parabolic shot always obeys the following considerations:

Examples of parabolic motion

1. The firing of a military projectile (artillery charge, mortar, etc.). From the barrel of the barrel to the drop point or target.
2. The kick of a soccer ball. From the archery to falling into the opposite field.
3. The path of a golf ball. During the initial long-distance shot.
4. The jet of water from a hose. Like those used by firefighters to put out a fire.
5. The jet of water from the rotating sprinklers. In a garden or park, throwing the liquid around it with a uniform speed and angle.
6. The throwing of a stone. When we try to knock down fruits from a tree but we miss them and they fall from the other side.
7. A volleyball serve. Which makes the ball rise above the net and land at the same angle of inclination on the other side.
8. Launching a bomb or missile. From an airplane in mid-flight, it is a semi-parabolic movement since it runs half a parabola (but responds to the same physical considerations).
9. The launch of a disc. Like those who jump to practice target shooting with a rifle.
10. The bounce of a stone on the surface of the water. It will draw smaller and smaller parabolas with each bounce, until it loses its initial thrust and sinks.

Examples of parabolic shooting exercises

1. Someone kicks a soccer ball, which is thrown at an angle of 37 ° and with a speed of 20 m / s. Knowing that the gravitational constant is 9.8 m / s ^ 2, calculate: a) the maximum height of the ball, b) the total time it remains in the air, c) the distance it has traveled when falling.

Resolution:

Vox = Vo Cos a = 20 m / s Cos 37 ° = 15.97 m / s

Voy = Vo Sen a = 20 m / s Sen 37 ° = 12.03 m / s

To get the maximum height time:

Vfy = 0 m / s (when it reaches the maximum height, vfy = 0)

Therefore: t = (Vfy – Voy) / g = (0 – 12.03 m / s) / (-9.8m / s²) = 1.22 s

to) To get the maximum height:

Ymax = I go t + gt² / 2 = 12.03 m / s (1.22 s) + ((-9.8m / s²) (1.22 s)²) / 2 = 7.38 m

b) To obtain the total time, simply multiply the maximum height time by 2, since we know that the trajectory in this case is symmetric: the projectile will take twice as long to fall as it did to reach its maximum height.

T_total = t_max (2) = 1.22s (2) = 2.44 s

c) To obtain the maximum range, the formula will be used:

x = v_x t_total = 15.97 m / s (2.44 s) = 38.96 m

v_fy = gt + v_hey = (- 9.8) (1 s) + 12.03 m / s = 2.23 m / s

v_fx = 15.97 m / s since it is constant throughout the motion.

2. An involuntary artillery fire occurs with a velocity of 30 m / s, forming an angle of 60 ° with respect to the horizon. To alert the civilian population, it is necessary to calculate (a) the total distance covered, (b) the maximum height and (c) the time of fall of the shot.

Resolution:

to) To get the distance traveled:

d = (v₀² sin α * cos α) / g = ((30m / s)² sin (60 °) * cos (60 °)) / 9.8 m / s² = 79.5 m

b) To obtain the height reached:

h = v₀²sen²α / 2g = (30 m / s)² sen² (60 °) / 2 (9.8 m / s²) = 34.44 m

c) To get the total time:

t = 2 * (v₀ sin α / g) = 30 m / s (sin 60 °) / 9.8 m / s² = 5.30 s